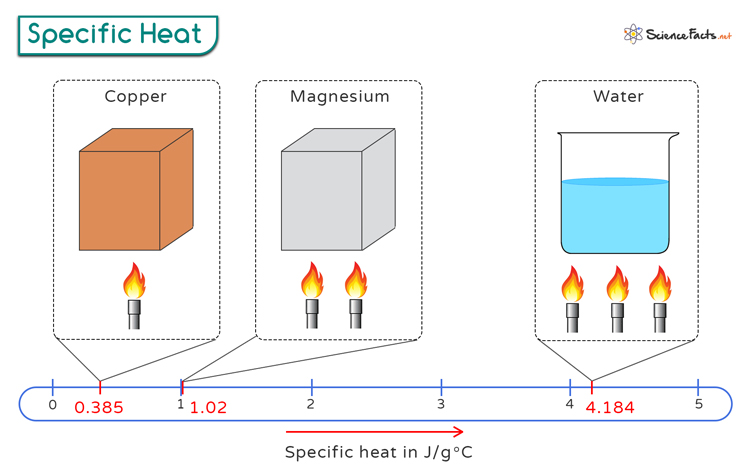
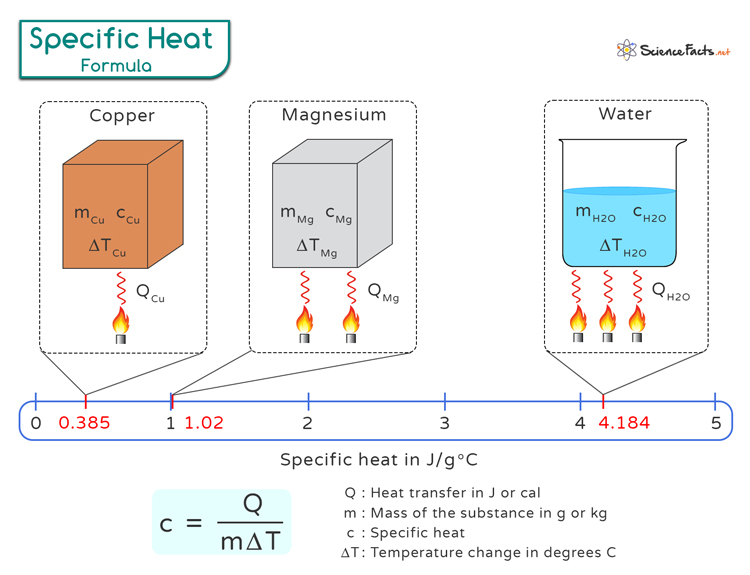
On the other hand, heat capacity is the amount of heat required to increase the temperature of the entire substance by one degree Celsius.
Specific Heat and Heat Capacity Formula
Specific Heat Table
Specific Heat of Water
Difference Between Specific Heat and Heat Capacity
Example Problems with Solutions
Where
Q represents the heat transfer in Joules (J) or calories (cal). m denotes the mass of the substance in grams (g) or kilograms (kg) being heated or cooled.c is the specific heatΔT represents the temperature change in degrees Celsius (∘C) of the substance
This formula assumes no phase change occurs during heating or cooling processes and applies specifically to substances with constant specific heat within a given temperature range. Rearranging the above equation, one can find the expression for specific heat. The heat capacity (C) can be calculated by multiplying the specific heat with the mass. Therefore, Units The unit of specific heat is Joules per gram per degree Celsius or J/g∙∘C. Another unit of specific heat is calories per gram per degree Celsius or J/cal∙∘C. The temperature change (∆T) in the Celsius (C) scale is the same as that in the Kelvin (K) scale, although the temperature values differ. Therefore, one can replace ∘C with K. In that case, the SI unit of specific heat is Joules per kilogram per degree Kelvin or J/kg∙K. The unit of heat capacity is J/∘C or cal/∘C. Molar Specific Heat The specific heat of a substance can also be described in terms of its molar amount. In that case, we use a term called molar specific heat. It is defined as the amount of heat required to change the temperature of one mole of a substance by one degree. It is represented in the unit of Joules per mole per degree Celsius or J/mol∘C. Water has a particularly high specific heat compared to many other substances. Its specific heat capacity is 4.184 J/g°C, which means it takes 4.184 Joules of energy to raise the temperature of 1 gram of water by 1 degree Celsius. Let us discuss the significance of this remarkable property of water. Thermoregulation is the process by which living organisms maintain their internal body temperature within a narrow range despite fluctuations in the external environment. In nature, bodies of water such as oceans, lakes, and rivers act as thermal regulators by absorbing excess heat during the daytime and slowly releasing it at night. This helps maintain stable temperatures in surrounding areas and supports diverse ecosystems. Understanding water’s specific heat is crucial for designing efficient heating and cooling artificial systems like car radiators and hot water pipes. Water’s ability to store large amounts of thermal energy makes it an ideal medium for transferring heat between different areas while minimizing temperature fluctuations. Solution: The heat transfer equation is given by: Heat lost by aluminum is: [ q_{\text{Al}} = m_{\text{Al}} \cdot c_{\text{Al}} \cdot \Delta T_{\text{Al}} ] [ q_{\text{Al}} = (200 , \text{g}) \cdot (0.9 , \text{J/g°C}) \cdot (40 , \text{°C} – 80 , \text{°C}) ] [ q_{\text{Al}} = -7200 , \text{J} ] The negative sign implies that aluminum loses heat to water. Therefore, the heat transferred to water is 7200 J. Problem 2: A 150 g piece of copper is heated from 20°C to 100°C. Calculate the heat energy absorbed by the copper. The specific heat of copper is 0.39 J/g∙°C. Solution: The heat transfer equation is given by: [ q = m \cdot c \cdot \Delta T ] [ q_{\text{copper}} = m_{\text{copper}} \cdot c_{\text{copper}} \cdot \Delta T_{\text{copper}} ] [ q_{\text{copper}} = (150 , \text{g}) \cdot (0.39 , \text{J/g°C}) \cdot (100 , \text{°C} – 20 , \text{°C}) ] [ q_{\text{copper}} = 4680 , \text{J} ] Therefore, the heat absorbed by copper is 4680 J.