Who Discovered Photoelectric Effect
Photoelectric Effect Explained
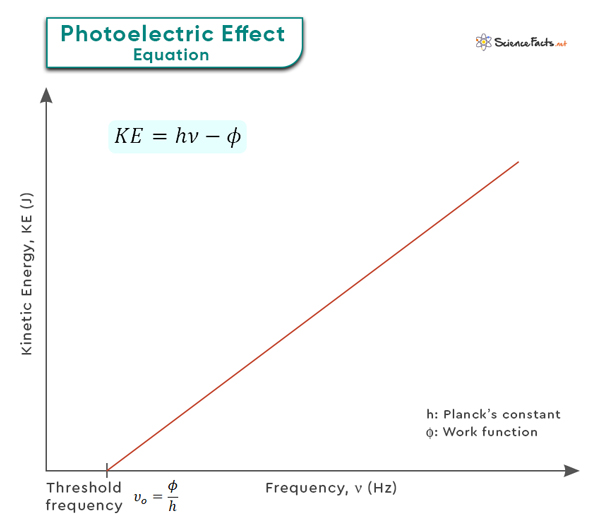
Equation for Photoelectric Effect
Work Function
Application
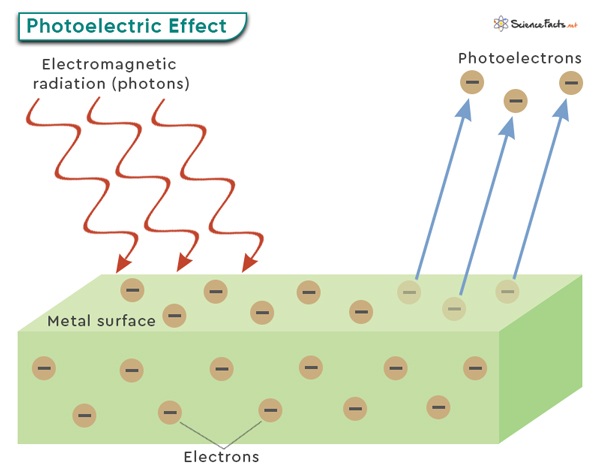
The photoelectric effect is typically observed with metals, as they have loosely bound or delocalized electrons that are more easily excited by incoming photons. However, it can also occur with other materials like semiconductors or insulators under specific conditions. A critical aspect of the photoelectric effect is that it follows specific principles. First, there is a minimum energy threshold that the incident photons must have to cause electron emission. This threshold energy is directly related to the material’s work function. Additionally, the intensity or brightness of the incident light does not affect the kinetic energy of emitted electrons but rather determines their number or intensity. On the other hand, changing the frequency or color of light can alter the kinetic energy of photoelectrons. E = φ + KE The components of this equation are as follows: By rearranging the above equation, one can determine the ejected electron’s kinetic energy. KE = hν – φ This equation emphasizes the direct relationship between the energy of incident photons and the photoelectron’s energy required to overcome the work function. It also demonstrates that electrons are only emitted when the energy of the incident photons is greater than the work function. We can also use this equation to find the photoelectron velocity (v), which is related to its mass and kinetic energy as follows: KE = ½ mev2 Therefore, ½ mev2 = hν – φ Where me is the rest mass of the electron (= 9.1 x 10-31 kg). Problem: What is the velocity of photoelectrons ejected from copper metal by an electromagnetic wave of frequency 4.0 x 1016 Hz? The work function of copper is 7.53 x 10-19 J. Given: ν = 4.0 x 1016 Hz, φ = 7.53 x 10-19 J We use the following equation: ½ mev2 = hν – φ => ½ x 9.1 x 10-31 kg x v2 = 6.62 x 10-34 Jˑs x 4.0 x 1016 s-1 – 7.53 x 10-19 J => v = 7.52 x 106 mˑs-1 Therefore, the electron moves with a velocity of 7.52 x 106 meters per second. Note that the kinetic energy of the photoelectron increases linearly with frequency as long as the photon energy is greater than the work function, precisely the relationship shown in the graph below. Different materials have different work functions, ranging from a few electron volts for metals to several for insulators. Materials with lower work functions are more susceptible to the photoelectric effect and emit electrons more readily when exposed to light. Understanding and manipulating the work function is essential for various applications. For example, solar cells should have a low work function at the electrode interfaces to facilitate efficient electron transfer. Additionally, controlling and optimizing the work function is crucial for achieving desired electrical properties in transistors and diodes.