Equation
Derivation
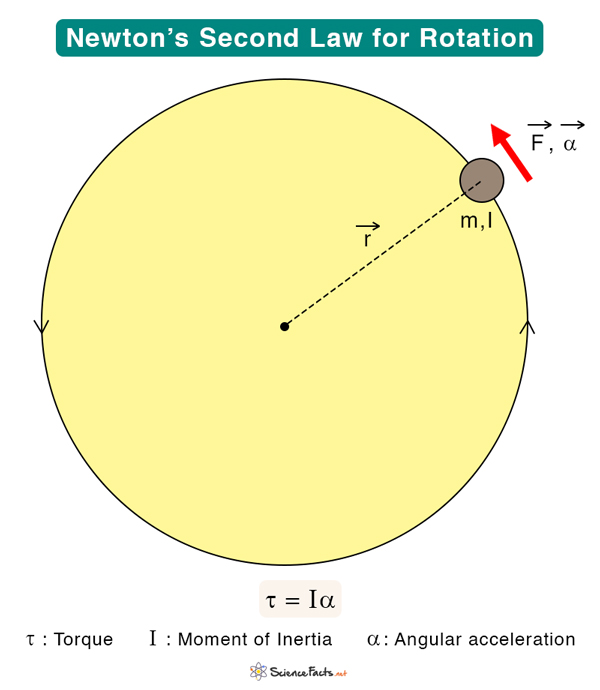
Where, τ: Torque I: Moment of inertia α: Angular acceleration The above equation is critical in rotational kinematics and tells us how torque, moment of inertia, and angular acceleration are related. The magnitude of linear acceleration in the direction of the force is The following equation relates the object’s tangential acceleration α to the linear acceleration. Substituting this into Newton’s second law, we obtain Multiplying both sides by r The left-hand side of the above equation is the torque τ, where r is the lever arm and F is the force perpendicular to r. Also, for a point particle, the moment of inertia is given by the following expression. Therefore, the applied torque on the object executing circular motion is If a rigid body experiences several torques, the net torque is the sum of all the torques, which equals the moment of inertia times the angular acceleration. The term Iα is scalar whose sign depends on whether the rotation is clockwise or counterclockwise. In vector form, Newton’s second law for rotation is given by