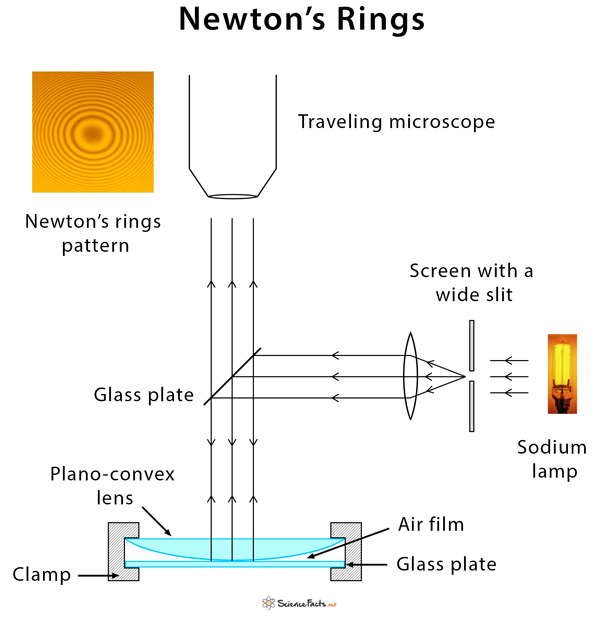
By studying the pattern of concentric rings, it is possible to determine the wavelength of monochromatic light and the refractive index of a given transparent liquid medium present in a wedge-shaped film. If white light is used instead of monochromatic, the lens system produces colorful interference, since the condition for a maximum in the interference is dependent on the wavelength. Newton’s rings have been named after English physicist and mathematician Sir Isaac Newton, who was the first to observe the effect in 1704.
An air film of varying thickness is formed between the lens and the glass sheet.When a ray falls on the surface of the lens, it is reflected as well as refracted.When the refracted ray strikes the glass sheet, it undergoes a phase change of 180° on reflection.Interference occurs between two waves that interfere constructively if the path difference between them is (m+1/2)λ and destructively if the path difference between them is mλ, thereby producing alternate bright and dark rings.
Nearly monochromatic source of light (sodium light)Plano-convex lensOptically flat glass platesConvex lensTraveling microscope
Method
The experimental setup for Newton’s ring is shown in the figure above. The convex surface of a plano-convex lens having a long focal length (large radius of curvature) is placed in contact with a plane glass plate and clamped together. Light from a monochromatic source (e.g., sodium lamp) is allowed to fall on a convex lens through a wide slit, which renders it into a nearly parallel beam. At first, light falls on a glass plate inclined at an angle of 45° to the vertical before reaching the lens-plate system at the bottom. Light is reflected from the upper surface of the glass plate and the lower surface of the lens. Due to the air film formed by the glass plate and lens, interference fringes are formed, which are observed directly through a traveling microscope. The rings are concentric circles. A dark central spot is obtained when viewed by reflection.
Theory and Equations
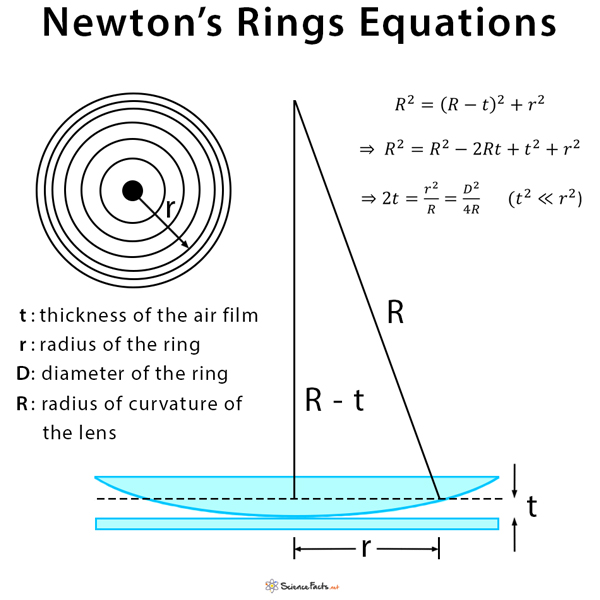
Consider a ray of light incident on the air film at a point where its thickness is t. The optical path difference between the two reflected rays will be 2t. Taking into account the phase change of 180° for reflection at the rare to the dense surface, the conditions for constructive and destructive interference are 2t = (m+1/2)λ (for constructive interference or bright rings) 2t = (m+1/2)λ (for destructive interference or dark rings) where m is the order of the ring and can take the values m = 0, 1, 2, 3, …, n If R is the radius of curvature of the lens and r is the distance of the point under consideration to the point of contact of the lens and glass plate, then R2 = (R-t)2 + r2 or, R2 = R2 – 2Rt + t2 + r2 or, 2t = r2/R = D2/4R since t2 « r2 and D = 2r, the diameter of a ring. Combine this result with the condition for the mth and nth dark rings. Then, the diameters of the two rings are given by Dm2 = 4Rmλ Dn2 = 4Rnλ Subtracting the two equations and rearranging Dn2 – Dm2 = 4R(n-m)λ λ = (Dn2 – Dm2)/4R(n-m) Thus, the wavelength λ can be determined from this equation. If a liquid of refractive index μ is introduced between the lens and the plate, then the path difference is given by 2μt. The diameter of any ring will be less with the liquid in place than without it. The equation for wavelength is given by λ = (Dn2 – Dm2)μ/4R(n-m) In a laboratory experiment, students are required to calculate the radius of curvature R of the lens. It is calculated by spherometer using the following relation R = l2/6h + h/2 Here, l is the mean length of the three sides of the equilateral triangle formed by joining the tips of three outer legs, and h represents the height of the central screw above or below the plane of the outer legs. Image courtesy: Softsolder.com
For testing the uniformity of a polished surface by studying the interference pattern the surface makes when placed in contact with a perfectly flat glass surfaceTo control the thickness of paint that is used on posters. Too much paint would exceed total weight requirements, and too little would result in faint imprints.