How to Calculate Moment of Inertia
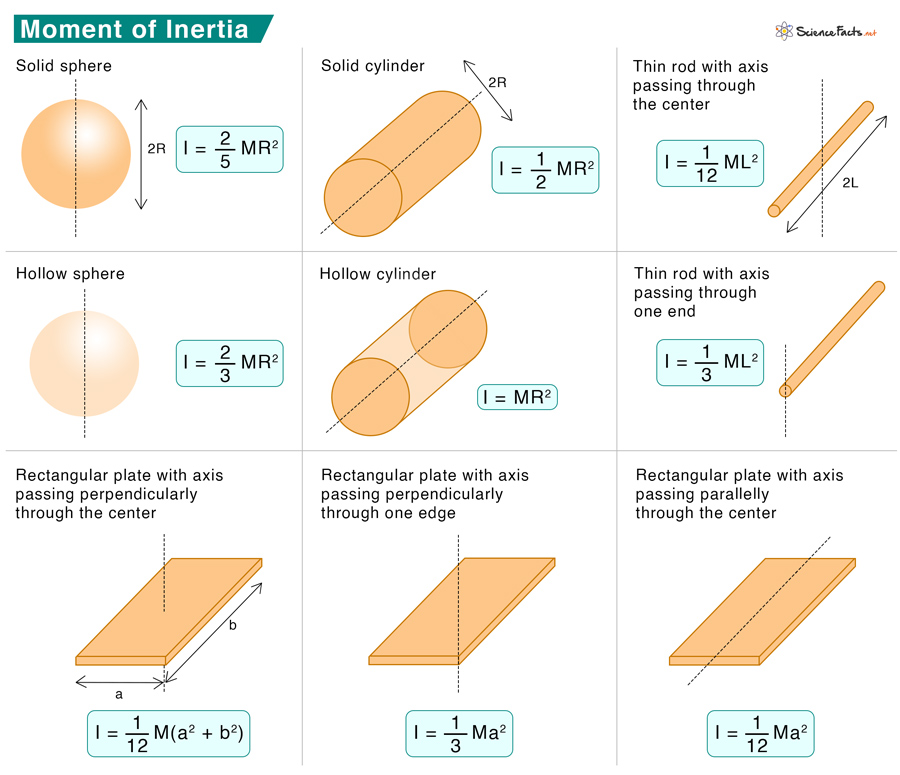
Moment of Inertia Table
Moment of Inertia and Rotational Kinematics
Factors Affecting Moment of Inertia
For a point mass (single body), the moment of inertia formula is given by the product of mass and the square of the object’s perpendicular distance from the axis of rotation. Where I is the moment of inertia m is the mass r is the perpendicular distance from the axis of rotation System of Particles Suppose n particles of masses m1, m2, …, mn rotate about a common axis at perpendicular distances r1, r2, …, rn from the axis. Then the moment of inertia of the system is The moment of inertia of a system of particles is the sum of the moments of inertia of the individual particles taken about a common axis. Units and Dimensions The SI unit of moment of inertia is kgˑm2, and the cgs unit is gˑcm2. The dimension is [M L2 T0].
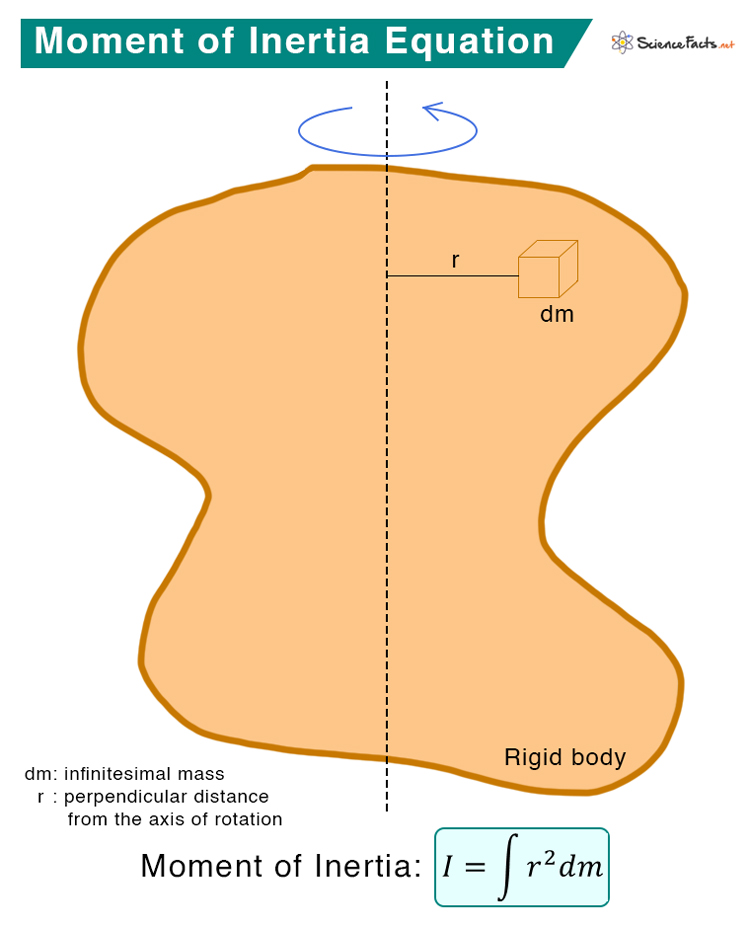
Integral Moment of Inertia
For a continuous mass distribution, the integral form of moment of inertia is given by Where dm is the instantaneous mass. The above equation can also be written in terms of density ρ and instantaneous volume dV as follows. Where τ is the applied torque I is the moment of inertia α is the angular acceleration The relationship between moment of inertia and rotational kinetic energy is given by And with angular momentum, the equation is Where K is the rotational kinetic energy L is the angular momentum ω is the angular velocity
The shape of the objectMass distribution or densityLocation of the axis of rotation