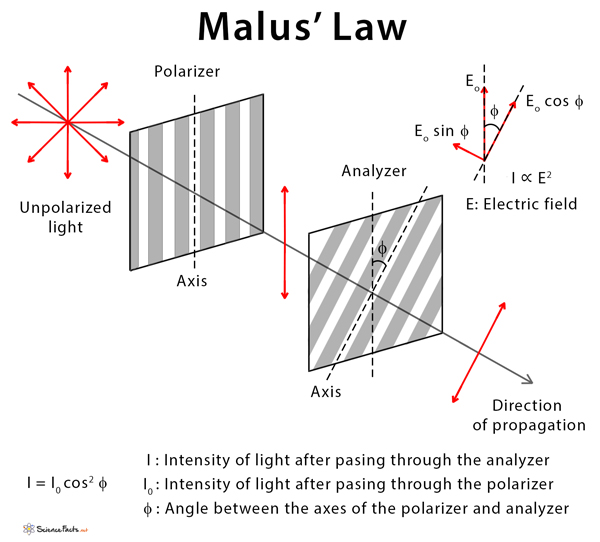
Malus’ law has been named after Étienne-Louis Malus, who was a French engineer, physicist, and mathematician. Malus published his work on the polarization of light in 1809. E = Eo cos Ø Where Eo is the amplitude of the electric field vector. This equation gives the displacement of the electric field vector after passing through the analyzer. Since the intensity, I, of the polarized beam is proportional to the square of the electric field, therefore, I ∝ E2 I = Io cos2 Ø This equation is Malus’ law. Note that, When Ø = 0° ( or 180° ), I = Io cos2 0° = Io. This condition implies that the intensity of light transmitted by the analyzer is maximum when the transmission axes of the analyzer and the polarizer are parallel to each other. When Ø = 90°, I = Io cos2 90° = 0. This condition implies that the intensity of light transmitted by the analyzer is minimum when the transmission axes of the analyzer and the polarizer are perpendicular to each other.