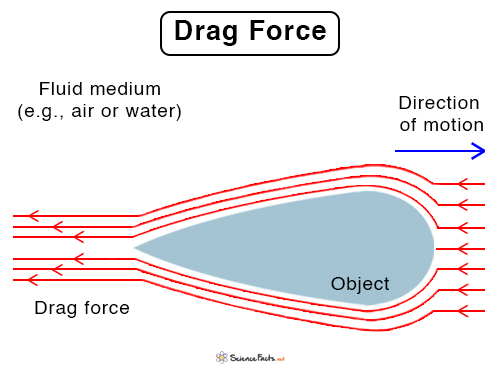
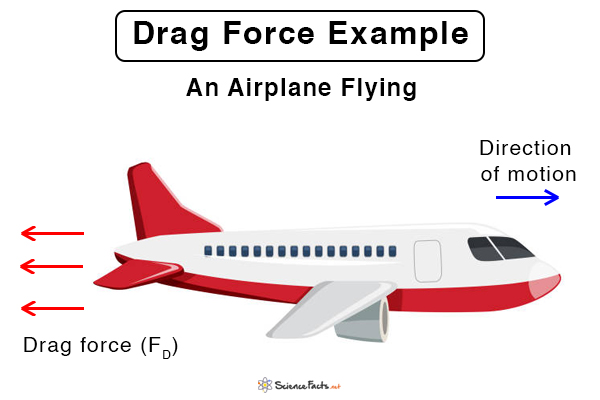
Examples of Drag Force
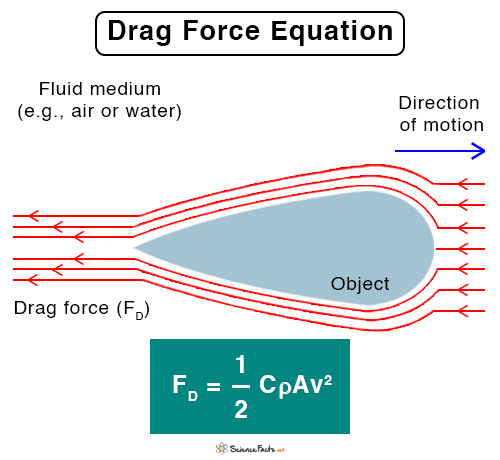
Drag Force Equation
Application of Drag Force
Lift and Drag Force
Drag Forces and Friction
A car driving on a highwayA ship sailing in the oceanA skydiver falling through the sky experiences drag after opening the parachute.A swimmer swimming in a poolA racing car speeding through a circuitAn airplane flying through the air
Where, C is the drag coefficient, which is dimensionless with values depending upon the shape of the object. (For example, C = 0.47 for a sphere and C = 0.82 for a long cylinder) ρf is the density of the fluid. v is the velocity of the object. A is the projected cross-sectional area of the object in a direction perpendicular to the object’s motion. Unit of drag force: Newton or N Dimension of drag force: MLT-2 The above equation applies to almost all fluids. As can be seen, FD is proportional to the square of the velocity, i.e., FD ∝ v2. This relationship is quadratic, and hence, the equation is also called quadratic drag force. The work done by the drag force per unit time, also known as power, is the product of FD times the velocity. P = FD x v
How to Find Terminal Velocity with Drag Force
When an object falls through the air from a high altitude, it experiences both gravity and drag force. Since gravity accelerates the object, its velocity will increase. Consequently, the drag force will increase and be equal in the magnitude of the gravity after some time. When it happens, there is no force acting on the object, which now moves through the air with a constant velocity. This velocity is known as terminal velocity vT and is derived as follows: At terminal velocity, v = vT. The drag force becomes And the force due to gravity is Where, m is the mass of the object g is the acceleration due to Earth’s gravity Since the two forces are equal, we have Viscous Drag Force The equation for FD does not apply in all situations. For viscous fluids, like honey and molasses, the drag force depends on the viscosity η. If the speed v is low (laminar flow), then the drag has a linear relationship with the velocity. For a spherical object of radius r, the magnitude of the drag force is given by Stokes equation. This kind of resistance where the liquid’s viscosity plays an important role is called the viscous drag force. Stokes equation is used to study small particles suspended in a liquid. Lift is a mechanical force generated by an object’s interaction with the fluid. Therefore, the presence of fluid is essential to generate a lift. This point is easy to understand because, on some planets and moons, where there is no atmosphere, it is practically impossible to lift and fly a plane. Unlike the drag force, the frictional force does not have a velocity dependence. This independence means that the force remains constant even if the object speeds up or slows down.